Unit-4
Design of Columns by Limit State Design Method
Q1) What are assumption for steel and concrete
A1)
ASSUMPTION
The assumptions for strength style of columns may be summarized as follows.
1. Strain of steel and concrete is proportional to distance from basic axis
2. Most usable compression strain of concrete is zero.
3. Stress, psi, in longitudinal reinforcing bars equals steel strain s times twenty nine,000,000 for strains below yielding, and equals the steel yield strength ƒ y, tension or compression, for larger strains
4. Durability of concrete is negligible.
5. Capability of the concrete in compression, that is assumed at a most stress of , should be in line with check results. An oblong stress distribution zero.85ƒc could also be used. Depth of the parallelogram could also be taken as a 1c, wherever c is that the distance from the neutral axis to the acute compression surface and one zero.85 for ƒ c 4000 psi and zero.05 less for every a thousand psi that ƒ c exceeds 4000 psi, however one mustn't be taken but zero.65.
Q2) Explain minimum and maximum eccentricity
A2)
Minimum Eccentricity (Clause 25.4 of IS456: 2000)
It is noted that in practical construction, columns are rarely truly concentric.
Even a theoretical column loaded axially which have the accidental eccentricity due to incorrect accuracy in construction or variation of materials etc.
Accordingly, all axially loaded columns which designed considering the minimum eccentricity which shows in Clause 25.4 of IS456 and given below.
E x min ≥ greater of (+) or 20 mm
E y min ≥ greater of (+) or 20 mm
Where l, D and b are the unsupported length, larger lateral dimension and least lateral dimension, respectively
Maximum Eccentricity (Clause 39.3 of IS456: 2000)
For the column to be short, as per Clause 39.3 the eccentricity shall not be more than 0.O5D or 0.05b.
Q3) Explain short column under axial compression write also steps to determine
A3) Assumptions (Clause 39.1 of IS456: (000)
The maximum compressive strain in form of the concrete in axial compression is taken as 0.002.
Strain in concrete and steel are equal.
The maximum compressive strain at the highly compressed extreme fiber which is form in concrete subjected to axial compression and bending and If is no tension on the section which is 0.0035 minus 0.75 times the strain at the least compressed extreme fiber.
Stress in steel is governed by characteristic stress-strain curve of steel in compression. The stress-strain curve of steel in compression is the same as in tension.
The column is short.
Equation for Short axially Loaded Tied Columns (Clause39.3 of IS456: 2000)
According to Clause 39.3 of IS456: 2000, when the eccentricity does not exceed 0.05 times the lateral dimension, the member may be designed by the following equation
P u = 0.4 f c k Ac + 0.67 f y A s c
Where,
P u = factored axial load on the member,
F c k= characteristic compressive strength where P. of the concrete,
A c =area of concrete,
F y= characteristic strength of the compression reinforcement, and
A s c = area of longitudinal reinforcement for columns.
Equation of Short Axially Loaded Columns with Helical Ties (Clause39.4 of IS 456: 2000)
The code further recommends that the ratio of volume of helical reinforcement to the volume of core shall not be less than 0.36 (Ag / Ac - 1) (f c k / f y), in order to apply the additional strength factor of 1.05 (Clause 39.4.1).
Accordingly, the governing equation of the spiral columns may be written as
P u = 1.05 (0.4 f c k Ac + 0.67 f y A s c)
= 0.4 f c k Ac + 0.67 f y A s c
The pitch of helical reinforcement is determined using the following relation as per CI 39.4.1 of IS456: 2000.
P <
Where,
Dc = Diameter of concrete core,
D s p = Diameter of spiral reinforcement,
A s p =Area of cross-section of spiral reinforcement,
p= Pitch of spiral reinforcement.
Note:
The pitch and diameter of the spiral reinforcement should also satisfy Clause 26.5.3.2 of 19456: 2000.
Design Procedure for Axially Loaded Short Columns
Step 1: Find effective length.
Step 2: Find the minimum eccentricity and check the type of column.
Step 3: Find the area of steel
Use, P u = 0.4 f c k Ac + 0.67 f y A s c
Step 4: Design of lateral ties
The diameter of transverse reinforcement (lateral ties) determined from Clause 26.5.3.2 C-2 of IS456 as not less a (1) d/2 (2) 6 mm.
Where, d = diameter of bar
The pitch of lateral ties, as per Clause26.5.3.2 C-1 of IS45 should be not more than the least of
The least lateral dimension of the column
Sixteen times the smallest diameter of longitudinal reinforcement bar which can tied.
300 mm
Step 5: Draw the cross section
Q4) Design an axially loaded column 400 mm x 450 mm pinned at both ends with an unsupported length of 3 m for carrying axial service load of 2000 kN. Use M20 Fe415.
A4)
Given:
b = 400 mm
D = 450 mm
L = 3m
P = 2000 KN
F c k = 20 N/mm2
F y = 415 N/mm2
To find: Reinforcements
Solution:
Step 1: Check for minimum eccentricity
e min = 21 mm or 20 mm ………….whichever is greater
e min = 21 mm
e max = 0.05 D = 0.05 x 450 = 22.5 mm
e min < e max , the formula given for axial load I applicable
Step 2: Factored load P u = d x p = 1.5 x 2000 = 3000 KN
Step 3: Longitudinal reinforcement:
P u = 0.4 f c k Ac + 0.67 f y A s c
Ag = 450 x 400 = 180000 mm2
Ac = Ag – A s c
= 180000 – A s c
3000 x 103 = 0.4 x 20 x (180000 – A s c) + 0.67 x 415 x A s c
= 1.44 x 106 – 8 A s c + 278.05 A s c
270 A s c = 1.56 x 196
A s c = 5777.8 mm2
Using 32 mm diameter of bar
Area of one bar Ad = • d2 = • 322 = 804.2 mm2
Number of bars n = 7.2 = 8 bar
Provide 8 – 32 mm bar as longitudinal steel
A s c provided = 8 x 322 = 6434 mm2
Pt = x 100
= x 100
= 3.6 % < 6 % ……………………OK
Use cover 40 mm
Steel spacing = 144 mm
Clear spacing between bars = 144 – 32 = 112 mm < 300 mm
Step 4: Transverse steel:
Diameter of link or lateral ties should not less not x diameter of largest longitudinal bar
= x 32 = 8 mm
Diameter should not less not 6 mm
D t = 8 mm ……………………..whichever is greater
Spacing for lateral ties
The least lateral dimension b = 400 mm
16 x diameter of longitudinal reinforcement bar = 16 x 32 = 512 mm
300 mm
Spacing = 300 mm ……….....whichever is least
Provided lateral ties of 8 mm diameter of bar at 300 mm c/c
Q5) What are requirements for reinforcement
A5)
Types of Column Reinforcement
A column has two types of reinforcements as mention as below,
Longitudinal or Main Reinforcement (Clause 26.5.3.1 of IS456: 2000)
The longitudinal reinforcement resists axial load and bending moment.
The transverse reinforcement resists shear force, shares a small fraction of axial load if provided in the form of helical reinforcement, keeps main reinforcement in position and prevents longitudinal bars from buckling.
The longitudinal reinforcing bars in the form of vertical steel are used to carry the compressive loads along with the concrete. The following are the IS requirement for design of column:
IS requirement for design of column
(A) Areas of longitudinal steel
Minimum amount of steel should not be less than 0.80 % of gross cross-sectional area
Amount of area should not be more than 4 % of the gross cross-sectional area of the column
In no case, it does not exceed 6 % when bars from column below have to be lapped with those in the column under consideration.
(B) Minimum Number of longitudinal steel
For rectangular column shall be 4
For circular columns shall be 6
(C) Diameter of longitudinal steel
The diameter of the longitudinal bars shall not be less than 12 mm
(D) Spacing of longitudinal bars
The minimum horizontal spacing between two parallel main bars shall not be less than diameter of larger bar or maximum size of coarse aggregate plus 5 mm.
The maximum spacing of longitudinal bars measured along the periphery of the column shall not be more than 300 mm.
(E) Nominal cover to longitudinal bars
Nominal cover in case of a column equals to (i) 40 mm (ii) the diameter of longitudinal bar whichever is greater.
In case of columns of width 200 mm or less whose longitudinal bar not exceed 12 mm diameter, the nominal cover of 25mm may be used.
Transverse or Lateral Reinforcement (Clause 26.5.3.2 of IS456: 2000)
Clause 26.5.3.2(b) of IS 456: 2000 stipulates the guidelines of the arrangement of transverse reinforcement as under.
As per Clause 26.5.3.2 (b)(1), If the longitudinal bars are not spaced more than 75 mm on either side, transverse reinforcement shall only go round corner and alternate bars for the purpose of providing effective lateral supports.
As per Clause 26.5.3.2 (b)(2),If longitudinal bars spaced at a distance not exceeding 48 times the diameter of the tie are effectively tied in two directions, additional open ties are provided in between longitudinal bars
As per Clause 26.5.3.2(b) (3),.For longitudinal bars in compression members are placed in more than one row
As per Clause 26.5.3.2(b) (3(i),Transverse reinforcement is provided for the outer-most row in accordance with (a) above, and
As per Clause 26.5.3.2 (b)(3(ii),no bar of the inner row is closer to the nearest compression face than three times the diameter of the largest bar in the inner row.
The diameter of such transverse reinforcement need not, however, exceed 20 mm
Pitch and Diameter of Lateral Ties (Clause 26.5.3.2(c) of IS456: 2000)
1) Pitch
The pitch of transverse reinforcement shall be the least of the following,
The least lateral dimension of the compression 9.5 members
Sixteen times the smallest diameter of the longitudinal reinforcement bar to be tied; and
300 mm.
2) Diameter
The diameter of the polygonal links or lateral ties is not less than one-fourth of the diameter of the largest longitudinal bar and in have no case less than 6 mm.
Q6) Explain short column under axial loads with uni axial bending
A6)
Design of Short Columns under Axial Load with Uni axial Bending
The design of columns is involves with the determination of percentage of longitudinal steel p, assuming or knowing the dimensions b and D, grades of concrete and steel, distribution of longitudinal bars in two or multiple rows and d'/D from the analysis or elsewhere. This can be minimised by using the design charts prepared by IS456 in SP-16.
The procedure to design the column using design charts of SP-16 is explained below:
Step 1: Assume the size of the column
Assume the size of the column and calculate d'/D.
Step 2: Calculate P u / f c k b D and M u / f c k b D2
Step 3: Selection of the design chart
Select suitable the design chart from SP-16 for the corresponding to d'/D, grade of concrete and distribution of steel.
Step 4: Determination of the percentage of longitudinal steel
Locate the point of intersection of P u /f c k b D and M u / f c k b D2 and find the suitable value of p/f c k
Thus, amount of longitudinal steel is obtained by using the following relation.
A s c = p b D /100 f c k
Step 5: Design of transverse reinforcement
Design the transverse ties as per the section 9.3.2.1,
Q7) Explain design of column under biaxial loading by design charts
A7)
DESIGN OF COLUMN UNDER BIAXIAL LOADING BY DESIGN CHARTS
IS Code Method for Design or Columns under Axial Load and Biaxial Bending (Clause 39.6 of IS456: 2000)
A Clause 39.6 of IS456 recommends the following Simplified formula, based on "Breslers load Contour Method", for the biaxial loaded columns. The relationship between Mu xx and Mu yz for a particular value of P u = Pu z expressed dimensional form is
(M u x / Mu x 1) + (M u y / M u y 1) ≤ 1
Where,
Mu x and Mu y= moment about x and y axes due to design load
Mux1and Mu y 1= maximum uni axial moments the column can take under the actual load P by bending about x and y axes.
n is related to P u,/P u z where
P u z= design load on the column
P u z= 0.45 f c k Ac + 0.75 f y A s c (I e value P u of when M = 0)
P u z= 0.45 f c k Ag (0.75 f y – 0.45 f c k ) A s c
n = Exponent whose value is to be taken as follow:
Where,
Ag= gross area of the section, and
A s c = total area of steel in the section
It is worth mentioning that the quantities Mu x , Mu y and P u are due to external loadings applied on the structure and are available from the analysis
Whereas Mu x , M u y and P u are the capacities of the column section to be considered for the design.
Solution of Problems using IS Code Method
18456: 2000, suggest the following steps to design the columns subjected to load and biaxial moment.
1) Selection of trial section for the design type of problems
The preliminary dimensions are assumed during the analysis of structure Assume the percentage of longitudinal steel from the given Mu x , Mu y, P u, f y and f c k
Note:
Pillai and Menon suggested a simple way of considering a moment of approximately 15 per cent in excess (lower percentage up to 5 per cent if P u/P u z is relatively high) of the resultant moment
Mu = 1.15 x (M u x 2 + M u y 2) .
As the uni axial moment for the trial section with respect to the major principal axis xx, if Mu x≥ Mu y otherwise, it should be with respect to the minor principal axis.
The reinforcement should be assumed to be distributed equally on four sides of the section.
2) Checking the eccentricities ex and ey for the minimum eccentricities
Clause 25.4 of IS256 stipulates the amounts of the minimum eccentricities and are given in Equation s follows,
(M u x /M u x 1)+ (M u y /M u y 1) ≤ 1
P u z= 0.45 f c k Ag + (0.75 f y – 0.45 f c k ) A s c
Where 1, b and D are the unsupported length, least lateral dimension and larger lateral dimension, respectively. The clause further stipulates that for the biaxial bending, it is sufficient to ensure that the eccentricity exceeding the minimum value about one axis at a time.
3) Verification of eccentricities
It is to be done determining ex = Mu x/P u and e y = Mu y/P u from the given data of Mu x, Mu y and P u and Equation above from the assumed b and D and given l eff.
4) Assuming a trial section including longitudinal reinforcement
This step is needed only for the design type of problem, which is to be done as explained in (a) above.
5) Determination of Mux1 and Muy1
Use of design charts should be made for this. Mux1 and Muy1 corresponding to the given P u should be significantly greater than Mu x and Mu y respectively.
6) Determination of P u z and n
The values of P u z and n can be determined from Equations as
P u z= 0.45 f c k Ag + (0.75 f y – 0.45 f c k )
. Alternatively, P u z can be obtained from Chart 63 of SP-16.
7) Checking the adequacy of the section
This is done either using Equation (9.16.1) or using Chart 64 of SP-16.
Design of isolated column footing for axial load and uni axial bending
Q8) Design an uni axial square, short column by limit state method with material M 25 and Fe 415 to carry ultimate load of 800 k N and working moment of 80 k Nm about major axis bisecting the depth of column. The unsupported length of column is 3.6m. The column is fixed at one end and hinged at the other. Also design the footing for this column only for flexure and punching shear. Take SBC = 250 k N/m2. Show detailed design calculations and reinforcement details in plan and sectional elevation
A8)
Given: Uni axial square column
M 25, f c k = 25 N/mm2
Fe 415 f y= 415 N/mm2
Ultimate load P = 800 k N
Working Moment M = 80 k Nm
Unsupported length L = 3.6 m
End condition: One end fixed and other hinged
Solution
A) Design of uni axial square column
Factored Load P u = 1.5 x P = 1.5 x 800 = 1200 k N
Mu = 1.5 x M = 1.5 x 80 = 120 k N m
Effective length for column Le = 0.8 L = 0.8 x 3600 = 2880 mm
Min. Size of short square column
L e / L L D1 ≤ 12
2880 / b ≤ 12
b > 240 mm
D = 400 mm
Eccentricity
Mu = P u x e
12 x 106 = 1200 x 103 e
e = 100m
e min = unsupported length/500 + lateral dimension /30
= 360/500 + 400/30
= 20.53 mm or 20 mm
e min = 20.5 mm
e > e min, the short column is designed for uni axial bending.
Determine d/D
Assume cover 60 mm, d/D =50 /400 = 0.15
Percentage of steel from design chart (refer sp – 16, chart 32)
Refer design chart for fy= 415 N/mm2 and d/D = 0.15
P u /f c k b D = 120 * 103 /25 * 400 *400 = 0.3
M u /f c k b D2 = 120 * 106 /25 * 400 * 4002 = 0.075
From chart, (refer chart 2 )
P u/ f c k = 0.03
P = 0.03 f c k = 0.03 x 25 = 0.75 %
Required percentage of steel is less than minimum percentage of steel as per IS 456 i.e. 0.8 %
Interaction Diagram for Combined Bending and Compression Rectangular Section-Equal Reinforcement on All sides .
Area of steel in compression
A s c = p b D /100 = 1 * 400 * 400 = 1600 mm2
Provided 4 bars of 20 mm and 4 bars of 16 mm
A s c provided = 4 * π/2 * 202 + 4 * π/2 * 162 = 2061 mm2> 1600 mm2
Design of lateral ties:
Diameter of link or lateral ties should not less not ¼ x diameter of largest longitudinal bar
= ¼ x 20 = 5 mm
Diameter should not less not 6 mm
D t = 6 mm ……………………..whichever is greater
Spacing for lateral ties
The least lateral dimension b = 400 mm
16 x diameter of longitudinal reinforcement bar = 16 x 20 = 320 mm
300 mm
Spacing = 300 mm ……….....whichever is least
Provided lateral ties of 6 mm diameter of bar at 300 mm c/c
Design of footing
Assume self wt. of footing = 10% of load on column
Total load on footing = 1.1 p = 1.1 x 800 = 880kN
Area of footing = total weight on footing /safe bearing capacity of soil
= P u /200 = 880/ 200
= 3.52 m2
Size of footing
Assume square footing L = B = 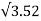 = 1.879 = 2 m
= 1.879 = 2 m
Provided size of footing = 2 m x 2 m
Since the column is subjected to eccentricity of 100mm, the C.G. of footing is taken at 100 mm away from the axis column as shown in fig as below,
Upward soil pressure = load on column /size of footing provided
= 800 /2 *2
= 200 KN/m2< SBC
Depth of footing based on BM:
Critical section for maximum bending moment occur at the face of column Projection beyond face of column
a = (B-d /2) + e = (2000 – 400/2 ) + 100
= 900 mm
Bending at face of column
BM = p o B a2 /2 = 200 * 2 * 0.92/2 = 162 K Nm
Factored BM,
Mu = 1.5 x M = 1.5 x 162 = 243 K Nm
For Fe415
Mu.lim = 0.138fck bd2
243 x 106= 0.138x 25 x 1000 x d2
d = 265.4 mm
Depth of footing based on punching shear:
Critical section for two way shear or punching shear is considered at a distance d/2 from the column face periphery.
Share width at critical Section b0 = b+ d = (0.4 + d)
Factored shear force
Vu= 1.5 p o (B2 - b02)
= 1.5 x 200 (22 - (0.4 + d2)
= 300 (4 – (0.16 + 0.8 d + d2)
= 300 (3.84 + 0.8d + d2) ………(1)
Shear capacity of concrete,
Vu = v (4 b o do)
But, 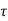 v= Ks
v= Ks 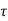 c = 1 x 0.25
c = 1 x 0.25 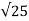 = 1.25 N/mm2
= 1.25 N/mm2
= 1250 KN/m2
= 500 (0.4 + d) d
= 500 (0.4d + d2) …………..(2)
Equating equation (1) and (2), we get,
300 (3.84 +0.8d + d2) = 500 (0.4d + d2)
3.84 +0.8 d + d2 = 16.67 (0.4d + d2)
3.84 +0.8d + d2 = 6.67d+ 16.67 d2
15.67d2 + 5.87 d - 3.84 = 0
Solving quadratic equation by using calculator,
d = 0.342 mm = 0.35 mm.
Assume cover to footing 0.5 m
Overall depth D = d +c = 0.35 + 0.5 = 0.4 m = 400 mm
Provide Depth for footing is 400 mm, which is greater value based on BM and punching shear.
Steel reinforcement
A s t = 0.5 f c k /f y (1 -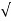 1 – ((4.6 M u)/ (f c k b d2) b d
1 – ((4.6 M u)/ (f c k b d2) b d
A s t = 2020.8 mm2
Using 12 mm diameter of bar
Area of one bar Ad = • d2 =
• d2 =  • 122 = 113.09 mm2
• 122 = 113.09 mm2
Number of bars n = A s t/ A d = 2020.8/ 113.09 = 17.9 = 18 bar
Provide 18 - 12 mm dia. of bars at an equal distance
Check for development length:
L d = 47 x d = 47 x12 = 564 mm
Q9) Design a bi-axial rectangular short column by limit state method with material M25 and Fe 415 to carry a working load of 900 k N. Working moment of 90 k N-m about major axis bisecting the depth of column and 35 k N-m about minor axis bisecting the width of column. The unsupported length of column about major and minor axis is 3.6 m and 3.2 m. The column is fixed at one end and hinged at the other. Show detailed design calculations and reinforcement details.
A9)
Given:
Working load = 900 k N
L ex= 3.6 m, f c k = 25 N/mm2
L e y = 3.2 m, f y = 415 N/mm2
Solution
Minimum eccentricities
Assume b = 300 mm, D = 500
E x min = greater of (3600/500+ 300/30 ) and 20 mm
= 17.2 mm and 20 mm
E y min = greater of (3200/500 +500/30) and 20 mm
= 23.06 mm and 20 mm
P u = ultimate load= 900 x 1.5= 1350 k N
Mu x = ultimate moment @ major axes
2x90 = 180 k N-m
Mu y= ultimate moment @major axes
2x35 = 70KN-m
E x = M u x/ P u = 133.33
E y = M u y/ P u = 25.93
ex and e y are greater than ex min and e y min
2 )Assuming trial section,
b = 300 mm, d = 500 mm
Mu = 1.15 (mu x2 + muy2) ½
= 1.15 (1802 + 702) ½
=222.10 K Nm
P u /f c k b D=1350 * 103 /25 *300 *500 = 0.36
M u/ f c k b D2= 222.10 * 106 /25 * 300 * 5002 = 0.12
d /D =50/ 500 = 0.1
= 0.08
P = 0.08fck = 0.08 x 25 = 2 %
Area of steel in compression
A s c =p b D/ 100 = 2 * 300 *500 /100 = 3000 mm2
Use 20 mm diameter of bar
Number of bar = 3000/ π/2 202 = 7.55 = 8
Provided 8 bar of 20 mm diameter of bar
A s c provided = = 8 x π/2 x 202 = 2513.3 mm2< 3000 mm2
Pt % (provided) =100 * 2513.3 /300* 500 = 1.68 %
3) Determine of Mu x 1 and Mu y 1
P u / f c k b D =1350 * 103 /25 * 300 * 500 = 0.36
p/ f c k = 1.68/25 = 0.0672
Determine,
M u /f c k b D2 = 222.10 * 10 6 / 25 * 300 * 500 2= 0.12
Mu x 1 = 0.11 x 25 x 300 x 5002
= 206.25 x 106 N mm > 180 N mm
P u /f c k b D = 0.36 and = 0.0672
d/b = 50 / 300 = 0.166
Determine value Muy1/ f c k b D2
d/b = 0.15 and d/b =0.2
For d/b = 0.15 = 0.11
For d/b = 0.2 = 0.09
For d/b = 0.166
Mu y 1/f c k b D2 = 0.09 + ((0.11 – 0.09)/(0.2 – 0.15)) x ( 0.2 – 0.166 ) = 0.104
Muy1 = 0.104 x 25 x 3002 x 500
= 117 x 106 KN m =117 KN m
As Mux1 and Muy1 are significantly greater than Mu x and Mu y respective . Redesign of section is not needed.
4) Determine P u z
P u z= 0.45 f c k Ag +( 0.75 f y – 0.45 f c k )A s c
= 0.45 x 25 x 300 x 500 + (0.75 x 415 -0.45 x 25 ) x 2513.3
= 2441.49 x 103 N
P u z = 2441.49 KN
5 ) Check for adequacy of section
(M u x/ Mu x 1)+ (M u y/ Muy1) ≤ 1
0.88 ≤ 1
Design is safe
Spacing for lateral ties
The least lateral dimension b = 300 mm
16 x diameter of longitudinal reinforcement bar = 16 x 20 = 320 mm
300 mm
Spacing = 300 mm ……….....whichever is least
Provided lateral ties of 8 mm diameter of bar at 300 mm c/c
Design of footing
Load of column = 900 KN
Assume self wt. of footing = 10% of load on column = 90 KN
Total load on footing = 900 + 90 = 990kN
Factored load = 1.5 x 990 = 1485 KN
Area of footing = factored load on footing/ ultimate bearing capacity of soil
= P u/200 = 1485 / 200 *2
= 3.71m2
Size of footing
Assume square footing L = B =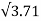 = 1.93 = 2 m
= 1.93 = 2 m
Provided size of footing = 2 m x 2 m
Upward soil pressure = load on column /size of footing provided
= 1.5 * 900/ 2* 2
= 337.5 KN/m2
Depth for BM
Determine eccentricity = e =M u/P u = 180/ (1.5 * 900) = 0.023 m = 23 mm
a1 = (L-b)/2 + e
= (2- 0.3)/2 + 0.023
= 0.873 m
a1 = (L-b)/2 - e
= (2- 0.3)/2 - 0.023
= 0.827 m
b1 = (B- a)/2
= (2- 0.5)/2
= 0.75 m
BM calculation
For BM calculation takes greater of a1 and a2
M x d = P u cal x a12/2 = upward soil pressure a12/2
= 337.5 x 0.8732 = 128.60 KN .m
My d = P u cal x b12/2 = upward soil pressure b12/2
= 337.5 x 0.752/2 = 95 KN .m
M d= maximum of M x d and My d= 128.60 KN m
For Fe415
Mu.lim = 0.138fck bd2
128.60 x 106= 0.138x 25 x 1000 x d2
d = 193.06 mm
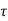 v = should be less than k s
v = should be less than k s  c
c
K s = 0.5 + 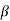 c = 0.5 +b/ D = 0.5 +300/ 500 = 1.1
c = 0.5 +b/ D = 0.5 +300/ 500 = 1.1
 c = 0.25
c = 0.25 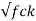 = 0.25
= 0.25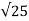 = 1.25 N/mm2
= 1.25 N/mm2
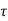 v = 1.1x 1.25 = 1.375 N/mm2
v = 1.1x 1.25 = 1.375 N/mm2
Nominal shear stress 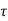 v = 1375KN/m2
v = 1375KN/m2
Vu = 2 [((2- 0.3)/ 2) – d ] x 337.5 ………1
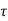 v < k s
v < k s 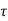 c
c
Shear resisted by concrete
Vu = 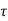 c B d
c B d
= 1375 x 2 x d
= 2750 d ……2
From equation 1 and equation 2
2 [ ((2- 0.3)/2 ) – d ] x 337.5 = 2750 d
573.75 – 675d = 2750d
d = 0.168m = 168 mm
Depth is safe against one way shear
Check for two way shear
Shear force on shaded area
Vu = [22 – (0.3 + d ) ( 0.5 +d) ]x 337.5…………Equation 1
Shear force resisted by concrete
Vu = 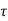 c b0 d
c b0 d
b0 = perimeter of critical section
b0 = 2 [(D + d) + (b + d)]
= 2 [(0.3 + d) + (0.5 + d)]
= 2[0.8 + 2d]
= 1.6 + 4d
Vu = b0 d
= 1375 (1.6 + 4d) d …………………Equation 2
Equating Equation (1) and (2)
[22 – (0.3 + d) (0.5 +d)] x 337.5= 1375 (1.6 + 4d) d
3.85 – 0.8 d – d2= 4.07 (1.6d + 4d2)
15.3 d2 + 7.31 d – 3.85 = 0
d = 0.316 = 0.316 m
= 320 mm
Overall depth = D = d + c + d/2
= 320 + 50 + 16/2
= 378 mm = 390 mm
Main reinforcement
A s t = 0.5 f c k /f y (1 -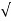 1 – ((4.6 M u)/ (f c k b d2) b d
1 – ((4.6 M u)/ (f c k b d2) b d
A s t = 1357.38 mm2
A s t for 2 m width = 2 x 1357.38 = 2714.77 mm2
Using 16 mm diameter of bar
Area of one bar Ad = π/2• d2 = π/2• 162 = 201.01 mm2
Number of bars n =A s t/ A d = 92714.77/ 201.01 = 13.5 = 14 bar
Provided 14 bars of 16 mm diameter of bar